Solutions and Hints
by Brent M. Dingle
for the book:
Precalculus,
Mathematics for Calculus 4th Edition
by James Stewart,
Lothar Redlin and Saleem Watson.
Problems
1.1:
1. 3x + 4y = 4y + 3x is demonstrating the commutative property of addition.
2. c(a + b) = (a+b)c is demonstrating the commutative property of multiplication.
3. (x + 2y) + 3z = x + (2y + 3z) is demonstrating the associative property of addition.
4. 2(A + B) = 2A + 2B is demonstrating the distributive property.
5. (5x + 1)3 = 15x + 3 is demonstrating the distributive property.
6. (x + a)(x + b) = (x+a)x + (x+a)b is demonstrating the distributive property.
Problems 7 through 12 rely mostly on the distributive property. All you need to do is multiply the problems out.
7. 3(x + y) = 3x + 3y (by distrib. prop.)
8.
(a – b)8 = a8
– b8 (by distrib. prop.)
=
8a – 8b (by comm. prop.)
9. 4(2m) = 8m (by distrib. prop.)
10.
(-6y) =
(
*(-6))y (by assoc.
prop.)
=
-8y (by distrib. prop.)
11.
(2x – 4y) = 
*2x - 
*4y (by distrib.
prop.)
=
-5x – (-10y) (by distrib. prop. twice)
=
-5x + 10y (prop. of numbers: minus
a negative becomes plus)
12.
(3a)(b + c – 2d) =
3ab + 3ac – (3a)(2d) (by distrib.
prop.)
=
3ab + 3ac – 6ad (by distrib.
prop.)
Problems 13 and 14 are just arithmetic operation problems and finding common denominators. Check you work with a calculator.
13.
a.
These have a common
denominator so just add the numerators
b.
Here you must find a common
denominator.
Notice
the Least Common Multiple of 10 and 15 is 30.
(10
* 3 = 30 and 15 * 2 = 30)
So
the common denominator you want to use is 30.
So
multiply 3/10 by 3/3 and multiply 7/15
by 2/2 gives:
and
you now have a common denominator so add
all
that remains is to add the numerators:
Notice
that 23/30 is a fraction in simplest form
(or
reduced form depending on the professor)
14. This is almost identical to 13.
a.
7/45 + 2/25. Notice A common denominator is 900 (there
are others)
this by 45 * 20 =
900 and 25 * 36. So solve as follows:
b.
5/14 – 1/21 + 1. Notice A common denominator is 42.
This by 14*3
= 42 and 21 * 2 = 42 and 1 * 42 = 42.
So we will
solve as follows:
Notice 55/42 is in Improper Form. Some professors will ask you to simplify this
to be a Mixed Fraction, which gives:
To do this you “take out” as many 42’s from 55 as possible. Since there is only
one 42 “in” 55 you get 1 as the integer part of the Mixed Fraction. Notice
after you “take out” 42 from 55 you have 13 left. The 13 becomes the numerator
of the fraction part. The denominator stays 42.
Problems 15 and 16 are just remembering that dividing by a fraction is the same as multiplying by its reciprocal (where the reciprocal is found by interchanging the top part with the bottom part of the fraction).
15.
a.
b.
This one is left for you to do. ( 15/2 = 7½ )
16.
a.
Notice that the
common denominator of
1/8 and 1/9 is
72 by 8*9=72 and 9*8 = 72.
So now we
subtract and multiply by the reciprocal of 1/72.
b.
This one we start for you but you must finish on your own:
For 17 through 20 you simply need to be good with number values. If in doubt check your answers with a calculator. An easy way to do this is to remember:
If a < b then a – b < 0.
So to see if –4.235 < -5.67 is true all you need to do is take
–4.235 – (-5.67) = 1.435
Since 1.435 is NOT less than zero than –4.235 < -5.67 is FALSE.
17. a) false b) true
18. a) true b) false
19. a) false b) true
20. a) false b) true
There isn’t much to 21 and 22.
21.
a) x > 0
b) t < 4
c) a ³
p
d) –5 < x < 1/3
e) | p – 3 | £
5 Notice distance is absolute
value, so p could be from 2 to 8
22.
a) y < 0
b) z > 1
c) b £
8
d) 0 < w £
17
e) | y - p
| > 2 Again distance is done in
absolute value.
For 23 to 26 remember union à A È B means stuff in either A OR B.
(The U for union)
And intersection à A Ç B means stuff in both A AND B
(the Çwhich looks kinda like N for aNd or maybe like an A for And)
Notice you are given:
A = { 1, 2, 3, 4, 5, 6, 7 } = { integers from 1 to 7 }
B = { 2, 4, 6, 8 } = { even integers from 1 to 8 }
C = { 7, 8, 9, 10 } = { integers from 7 to 10 }
23.
a) A È B = stuff in either A OR B = { 1, 2, 3, 4, 5, 6, 7, 2, 4, 6, 8 }
and we remove the
duplicates = { 1, 2, 3, 4, 5, 6, 7, 8 }
b) A Ç
B = stuff in both A AND B = { 2, 4, 6 }
notice this
corresponds to the duplicates
24.
a) B È C = B or C = { 2, 4, 6, 8,
7, 8, 9, 10 } = { 2, 4, 6, 7, 8, 9, 10 }
b) B Ç
C = B and C = { 8 }
25.
a) A È C = {1, 2, 3, 4, 5, 6, 7,
7, 8, 9, 10} = { 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 }
b) A Ç
C = { 7 }
26.
a) A È B È C = { 1, 2, 3, 4, 5, 6, 7, 2, 4, 6, 8, 7, 8, 9, 10 }
= { 1, 2, 3, 4,
5, 6, 7, 8, 9, 10 }
b) A Ç
B Ç
C = { } = Æ
= empty set.
This is because there is no number
which appears in all three sets.
You can also look at it this way:
A Ç B Ç C = (A Ç B) Ç C =
{ 2, 4, 6 } Ç
C
and
{ 2, 4, 6 } Ç C =
{ 2, 4, 6 } Ç
{ 7, 8, 9, 10 } = { }
For 27 and 28 you are given:
A = { x | x ³ -2 } ß
read that as: The set of x, such that x is greater than or equal to –2.
B = { x | x < 4 }
C = { x | -1 < x £ 5 }
You are to find the indicated set.
27.
a) B È C = set of x such that x is less than 4 OR x is > -1
and £
5
notice anything
between –1 and 4 is less than 4 – so the
sets overlap there.
Consider the picture:
thus if we combine everything covered
by the gold arrow and the green
line segment we get:
B È
C = { x | x £
5 }
b) B Ç
C = set of x such that x is less than 4 AND x > -1 and x £ 5.
And from the above picture we see
that the gold and green OVERLAP
from –1 to 4 (non-inclusive), i.e.:
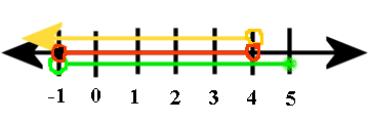
thus
B Ç
C = { x | -1 < x < 4 }
28.
a) A Ç C = set of x such that x ³ -2 and x > -1 and x £ 5
since x > -1 gives
us that x ³
-2 we can simply say
= set of x such that x
> -1 and x £
5
= { x | -1 < x £ 5 }
b) A Ç
B = set of x such that x ³ -2 and x < 4
= { x | -2 £ x
< 4 }
For 29, 31 and 33 see the back of your book. These are pretty straight forward.
Remember:
1. the parenthesis means ‘open circle’ (or just less than or greater than) and
2.
the square bracket means ‘closed circle’ (or dot),
i.e. less than equal to ( £ ) or
greater than equal to ( ³ )
29.
(-3, 0) --- see your book
30.
(2, 8]: In inequality
terms is 2 < x £
8
31.
[-2, 8) --- see your book
32.
[-6, -1/2]: In inequality terms is –6 £ x £ -1/2
33. [2, ¥) --- see your book
34.
(-¥,
1) : In inequality terms is -¥ < x < 1
For 35, 37, 39 see your book. These again are straight forward.
Remember
a. < or > means open circle or parenthesis
b. £ or ³ means closed circle or square bracket
35. x £ 1 --- see your book
36.
1 £
x £
2: In interval notation is [1, 2]
37. –2 < x £ 1 --- see your book
38.
x ³
-5: In interval notation is [-5, ¥)
39. x > 1 --- see your book
40.
–5 < x < 2: In
interval notation is (-5, 2)
Problems 41 through 46 should be simple enough if you have done the above problems.
For 41, 43, 45 see your book.
41. (-2, 0) È (-1, 1) --- see your book
42. (-2, 0) Ç (-1, 1) --- left for you to figure out ( try (-1, 0) )
43. [-4, 6) Ç [0, 8) --- see your book
44. [-4, 6) È [0, 8) --- left for you to figure out ( try [-4, 8) )
45. (-¥, -4) È (4, ¥) --- see your book
46.
(-¥,
6] Ç
(2, 10)
Consider the picture and notice the overlap:
And you should see the intersection is
an OPEN CIRCLE on 2
a CLOSED CIRCLE on 6
and a line between 2 and 6.
47. a) 100 b) 73
48.
a) 
(abs. val. always is positive so
subtract the smaller value from the larger)
b) |10 - p|
= 10 - p
»
6.858407346
49.
a) | |-6| - |-4| | =
| 6 – 4 | = | 2 | = 2
b) –1 / |-1| = -1 / 1 = -1
50.
a) | 2 - |-12| | = |
2 – 12 | = | -10 | = 10
b) –1 - | 1 - |-1| | = -1 - | 1 – 1 |
= 1 - | 0 | = 1 – 0 = 1
51.
a) | (-2)*6 | = | -12
| = 12
b) | (-1/3)*(-15) | = | 5 | = 5
52.
a) | - 6 / 24 | = |
-1 / 4 | = ¼
b) 
For 53 and 54 remember distance means absolute value.
(Have you ever gone –5 feet ?) Later you will see negative signs can be used to represent the direction you are moving, but the absolute value always represents the DISTANCE.
53.
a) |2 – 17| = |17 – 2| = 15
b) |-3 – 21| = |21 – (-3)| = 24
c) |11/8 – (-3/10)| = | -3/10 – 11/8 | = 67/40
54.
a) notice you can use 210 as the common denominator
15*14 = 210 and 21 * 10 = 210
| 7/15 – (-1/21) | = | -1/21 – 7/15
| = 48/210 = 18/35
b) | -38 – (-57) | = | -57 – (-38) | =
19
c) |-2.6 – (-1.8) | = | -1.8 – (-2.6) |
= 0.8
55.
a) 
è 10x =
7.7777777777… ( choose
10x as the full repeat ends in the tenths place)
1x =
0.7777777777… (1x as
there are NOT ANY non-repeating
decimals)
------------------------------- (subtract the second equation from the first)
9x = 7
x = 7/9
Thus we have:
b) 
è 100x = 28.8888… (choose 100x as full repeat ends in hundredths
place)
10x = 2.8888… (10x as the non-repeating decimals ended in
the tenths place)
------------------------------ (subtract the second equation from the first)
90x =
26
Thus we have:
c) 
è
100x = 57.575757… (choose 100x as full repeat
ends in hundredths place)
1x = 0.575757…
(1x as there are
0 non-repeating decimals, now subtract the eqs.)
---------------------------------
99x =
57
So we have:
56.
a) 
è 100x =
523.232323… (choose
100x as full repeat ends in hundredths place)
1x = 5.232323… (1x as there are 0 non-repeating decimals)
------------------------------- (subtract the second equation from the first)
99x =
518
So:
Notice you could have also just
figured out that 
and say 
= 5 + 
= 5 + 
= 5
b)
è 100x =
137.77777… (choose 100x
as full repeat ends in hundredths place)
10x =
13.77777… (10x
as the non-repeating decimals ended in the tenths place)
------------------------------- (subtract the second equation from the first)
90x =
124
So:
c) 
è 1000x
=
2135.3535… (choose
1000x as full repeat ends in thousandths place)
10x = 21.3535… (10x as the non-repeating decimals ended in
the tenths place)
------------------------------- (subtract the second equation from the first)
990x =
2114
So:
57.
In this problem you are given the inequality:
, where x = city miles and y = highway miles
a) For this part all you need to do is
plug in the numbers for x and y
and see if the inequality stays
true:
Since 12.65756 £ 15
the inequality stays true
then the car will NOT run out of
gas.
b) In this part you know the car has been driven 280 miles in the city.
So x = 280. To solve this you put
280 in for x. As you want to know the
number of miles it can be driven
before it runs out of gas you need to
find the y value that will make the
inequality EQUAL 15 (you want to
use up all 15 gallons of gas). So
you solve the following equation for y:
The first thing to do is simplify 1/28
* 280
Now subtract the 10 to the other side.
And
multiply both sides by 34 gives:
y = 170 miles
So the car can be driven 170 miles
on the highway before running out
of gas (if it was already driven 280
miles in the city).
No answers for 58, 59, 60 and 61 will be given here. They are better left for you to think out.
As a hint for 57 and 58 consider that 
. So you have an irrational times an irrational equaling a
rational. So it might be said that the irrationals are a larger set than the
rationals. More specifically which set is a subset of the other?